When Is A Triangle Not A Triangle ?

The overlap of three circles as shown below forms the simplest polygon of constant width : a reuleaux triangle.

Useful if you are designing coins to allow use in vending machines alongside more conventional circular coins.

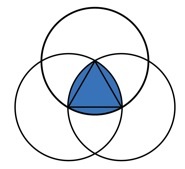
Or a little closer to home - a guitar pick with a symetrical shape - easier to orient in the hand without stopping to look.

What form can possibly replace the circle for a manhole cover without falling in to the manhole itself should it be misaligned ?

Shown here in the streets of San Fransisco.

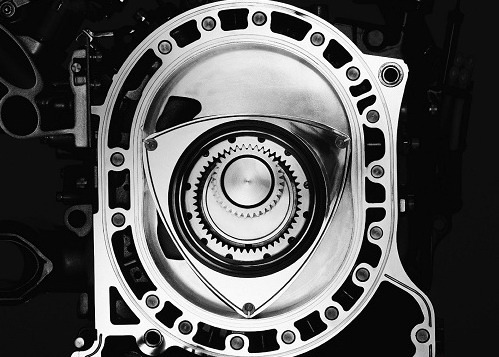
And with slight modification the geometric simplicity and strength of the Reuleaux Triangle forms the piston for the Wankel Rotary engine - patented in the 1920s and perfected in post war Germany. These engines provided a soundtrack to my youth in the Australian suburbs, the bark of little mazda sedans climbing the Brisbane hills and seemingly never changing gears ...

Not leading anywhere particularly useful in guitar design today - other than a lovely shape for some control covers with symmetry in more than one axis and echoes of past engineering conundrums.

One of my favourite tangents to this story is the development by a small US toolmaker, ( still extant and manufacturing without a website ) Watts Brothers Toolworks of Wilmerding, Pennsylvania of the square drill assembly. 

If a cutting tool in the form of a the realeaux triangle is allowed to rotate and it's 'centre' to move freely within the constraints of a hollow shaft ( a 'floating' chuck ) it will trace the outline of a perfect square ( give or take the fine points at each corner. )

I always knew such tools existed but didn't realise the connection to the Reuleaux Polygon until recently.

And finally to illustrate what a shape of constant width can do when extrapolated into the third dimension - here's a 3 dimensional version : as the reuleaux triangle is to the circle, this form is to the sphere, having less mass but providing constant support.

AP